Inverse Property In Math
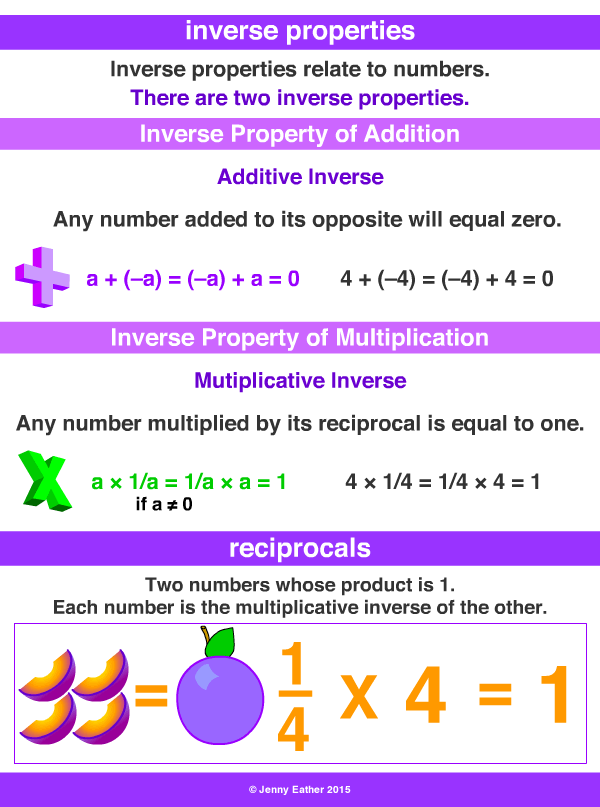
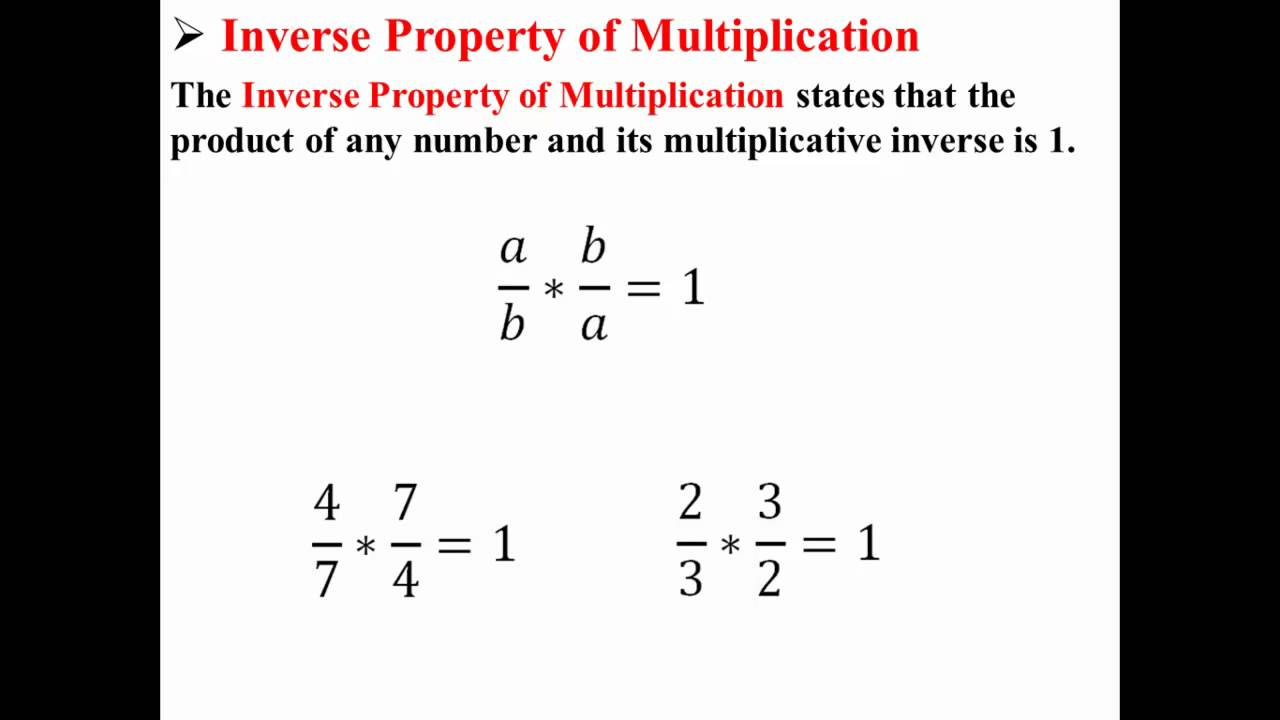
Inverse Property In Math - Use the inverse properties of addition and multiplication; What is the inverse property? Multiplying a number by its reciprocal (the multiplicative inverse) is always. Recognize the identity properties of addition and multiplication; Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. Illustrated definition of inverse property of multiplication: The inverse property says that, for a given number (and operation), there is another number which will take the.
Illustrated definition of inverse property of multiplication: The inverse property says that, for a given number (and operation), there is another number which will take the. Use the inverse properties of addition and multiplication; Recognize the identity properties of addition and multiplication; What is the inverse property? Multiplying a number by its reciprocal (the multiplicative inverse) is always. Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a.
What is the inverse property? Recognize the identity properties of addition and multiplication; Multiplying a number by its reciprocal (the multiplicative inverse) is always. The inverse property says that, for a given number (and operation), there is another number which will take the. Illustrated definition of inverse property of multiplication: Use the inverse properties of addition and multiplication; Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a.
PPT Do Now 9/21/10 PowerPoint Presentation, free download ID3579636
Use the inverse properties of addition and multiplication; Illustrated definition of inverse property of multiplication: Multiplying a number by its reciprocal (the multiplicative inverse) is always. What is the inverse property? Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a.
inverse properties A Maths Dictionary for Kids Quick Reference by
Recognize the identity properties of addition and multiplication; Illustrated definition of inverse property of multiplication: The inverse property says that, for a given number (and operation), there is another number which will take the. What is the inverse property? Multiplying a number by its reciprocal (the multiplicative inverse) is always.
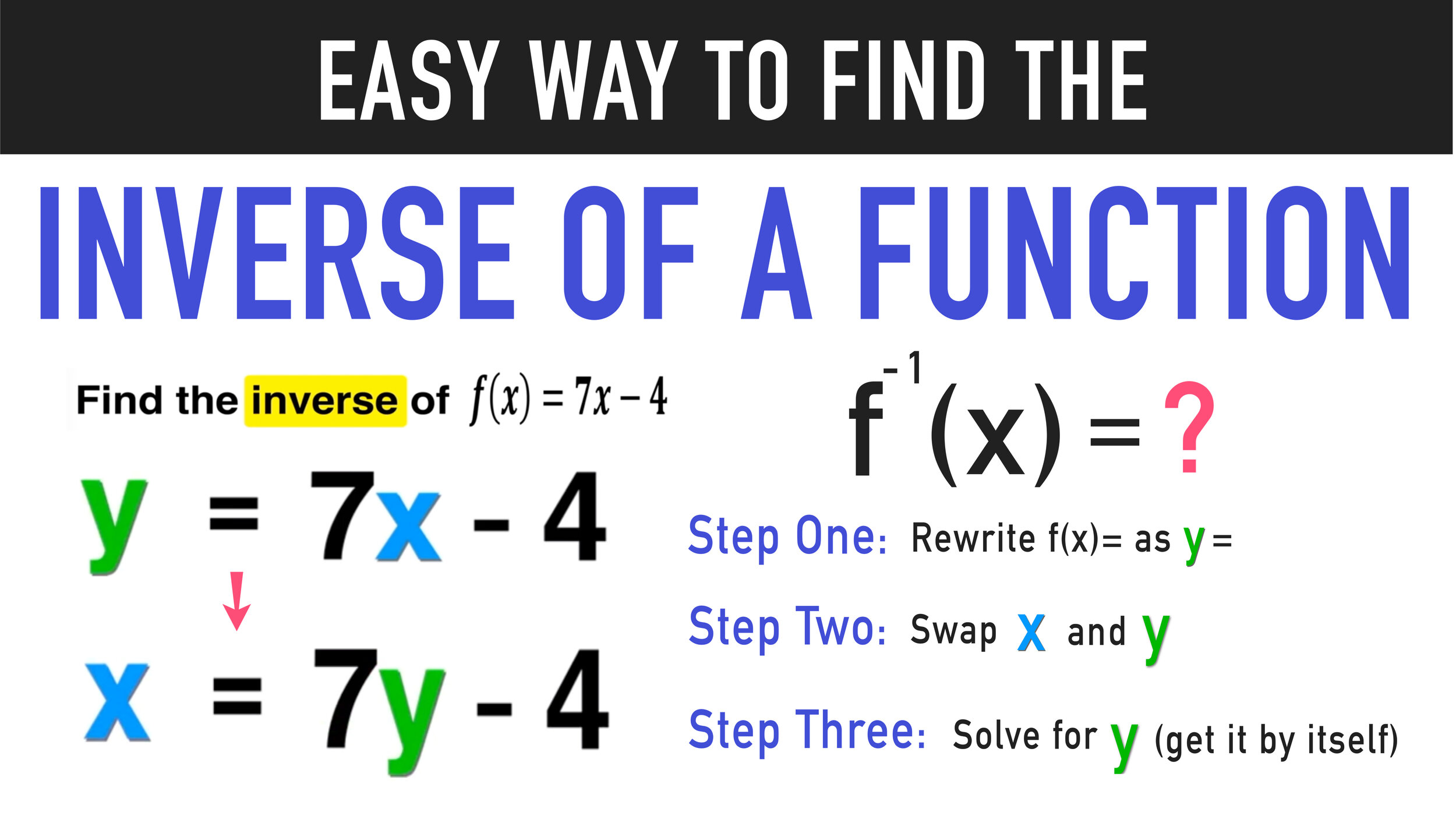
How to Find the Inverse of a Function 4 Steps (with Pictures)
Multiplying a number by its reciprocal (the multiplicative inverse) is always. Use the inverse properties of addition and multiplication; The inverse property says that, for a given number (and operation), there is another number which will take the. Recognize the identity properties of addition and multiplication; Inverse property of addition for any real number a, \[a + (−a) = 0\].
Additive Inverse Examples
Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. The inverse property says that, for a given number (and operation), there is another number which will take the. What is the inverse property? Illustrated definition of inverse property of multiplication: Use the inverse properties of addition and multiplication;
Distributive Property Equation Inverse Operations
Multiplying a number by its reciprocal (the multiplicative inverse) is always. What is the inverse property? The inverse property says that, for a given number (and operation), there is another number which will take the. Recognize the identity properties of addition and multiplication; Use the inverse properties of addition and multiplication;
Multiplicative Inverse Property Worksheets Free Printable
Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. Multiplying a number by its reciprocal (the multiplicative inverse) is always. Use the inverse properties of addition and multiplication; The inverse property says that, for a given number (and operation), there is another number which will take the. Recognize.
Télécharger l'image image addition 293660Image addition and
Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. Use the inverse properties of addition and multiplication; Multiplying a number by its reciprocal (the multiplicative inverse) is always. The inverse property says that, for a given number (and operation), there is another number which will take the. Recognize.
Inverse Property of Addition and Multiplication 1 YouTube
Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. Multiplying a number by its reciprocal (the multiplicative inverse) is always. What is the inverse property? Illustrated definition of inverse property of multiplication: Use the inverse properties of addition and multiplication;
Finding the Inverse of a Function Complete Guide — Mashup Math
Illustrated definition of inverse property of multiplication: The inverse property says that, for a given number (and operation), there is another number which will take the. Use the inverse properties of addition and multiplication; What is the inverse property? Recognize the identity properties of addition and multiplication;
Inverse Property of Addition & Multiplication Opposites & Reciprocals
Recognize the identity properties of addition and multiplication; Use the inverse properties of addition and multiplication; Illustrated definition of inverse property of multiplication: What is the inverse property? Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a.
The Inverse Property Says That, For A Given Number (And Operation), There Is Another Number Which Will Take The.
Use the inverse properties of addition and multiplication; Inverse property of addition for any real number a, \[a + (−a) = 0\] −a is the additive inverse of a. What is the inverse property? Illustrated definition of inverse property of multiplication:
Recognize The Identity Properties Of Addition And Multiplication;
Multiplying a number by its reciprocal (the multiplicative inverse) is always.